Master Statistics and Probability Problems with Expert Help
villain hero2023-01-11A student is asked to pick marbles from a bag that contains 18 marbles. 8 marbles are red, 4 are green, and 6 are black. What is the minimum number of marbles that a blindfolded student would have to draw from the bag to be certain of having at least three marbles of red color?
A student is asked to pick marbles from a bag that contains 18 marbles. 8 marbles are red, 4 are green, and 6 are black. What is the minimum number of marbles that a blindfolded student would have to draw from the bag to be certain of having at least three marbles of red color?
Linda Norman 2023-01-09Who discovered and first used the constant J.s?
1) Bohr
2) Einstein
3) De Broglie
4) Planck
1) Bohr
2) Einstein
3) De Broglie
4) Planck
Kaitlin Jacobson 2022-12-19How to evaluate the following sum?
What I have tried:
Above sum can be written as
What I have tried:
Above sum can be written as
sestigtalill 2022-12-19Geometric distribution. Johnny has 1,176 Pok´emon cards in total. Pok´emon EX is a special type of card, and Johhny has 39 EX-type cards. He is looking for an EX-type card, but all of the cards are completely mixed up and stored in a shoe box. His mother is calling him for dinner. What is the probability that Johnny will have to look through no more than 25 cards before he finds an EX-type card?
Amiya Hart 2022-12-18Is the correct statement that the data are discrete because the data can only take on specific values.
Tanja R.2022-12-13A Finite final consists of 50 multiple choice questions. If each question has 5 choices and 1 right answer, find the probability that a student gets an A (i.e. 45 or better) by purely guessing on each question.
A Finite final consists of 50 multiple choice questions. If each question has 5 choices and 1 right answer, find the probability that a student gets an A (i.e. 45 or better) by purely guessing on each question.
Juan Gonzalez2022-12-12Assume that when adults with smartphones are randomly selected, 48% use them in meetings or classes. If 25 adult smartphone users are randomly selected, find the probability that exactly 16 of them use their smartphones in meetings or classes.
Assume that when adults with smartphones are randomly selected, 48% use them in meetings or classes. If 25 adult smartphone users are randomly selected, find the probability that exactly 16 of them use their smartphones in meetings or classes.
Juan Gonzalez2022-12-12Assume that when adults with smartphones are randomly selected, 48% use them in meetings or classes. If 25 adult smartphone users are randomly selected, find the probability that exactly 16 of them use their smartphones in meetings or classes.
Assume that when adults with smartphones are randomly selected, 48% use them in meetings or classes. If 25 adult smartphone users are randomly selected, find the probability that exactly 16 of them use their smartphones in meetings or classes.
Cece Adonai2022-12-11
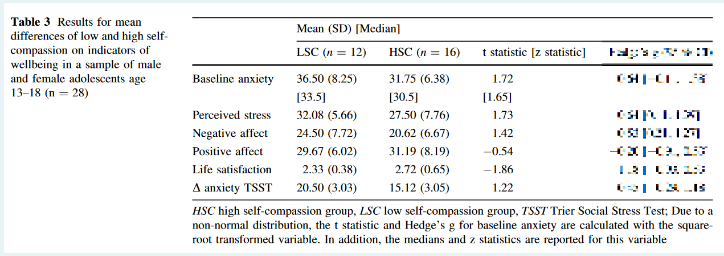
Which of the following statements is correct?
a.) With a larger sample, the mean differences examined here would increase (all other parameters remaining the same).
b.) If the standard deviations of the variable "Δ anxiety TSST" in both groups were smaller than those reported here, the t-value for the mean comparison of these variables would be larger (all other parameters being equal).
c) If, in addition to male and female adolescents, adolescents of diverse genders had also been interviewed, the t-test would no longer have been applicable. i
.e.) If it turned out that some questionnaires were incorrectly scored and the mean values for perceived stress in the group of high self-compassion were greater than 27.50, this would increase the t-value for the comparison of means (all other parameters being equal ). e.
d) To compare negative and positive affect within the group of people with low self-compassion, a one-sample t-test would be used.
Which of the following statements is correct?
a.) With a larger sample, the mean differences examined here would increase (all other parameters remaining the same).
b.) If the standard deviations of the variable "Δ anxiety TSST" in both groups were smaller than those reported here, the t-value for the mean comparison of these variables would be larger (all other parameters being equal).
c) If, in addition to male and female adolescents, adolescents of diverse genders had also been interviewed, the t-test would no longer have been applicable. i
.e.) If it turned out that some questionnaires were incorrectly scored and the mean values for perceived stress in the group of high self-compassion were greater than 27.50, this would increase the t-value for the comparison of means (all other parameters being equal ). e.
d) To compare negative and positive affect within the group of people with low self-compassion, a one-sample t-test would be used.
Cassius Delacruz 2022-12-05Find the values of x for which the series converges.
Find the sum of the series for those values of x.
Find the sum of the series for those values of x.
NormmodulxEE 2022-12-03Find the average value of the function f on the given interval.
geoffery mumali2022-11-29The joint cumulative density function of the random variables X and Y is given by:
Find the:
(i) Joint probability density function of the random variables X
and Y. [3 marks]
(ii) Marginal density function of X [3 marks]
(iii) Marginal density function of Y [3 marks]
(iv) Conditional density function of X given Y = y. [2 marks]
(v) Conditional density function of Y given X = x. [2 marks]
(vi) Are X and Y independent? [2 marks]
The joint cumulative density function of the random variables X and Y is given by:
Find the:
(i) Joint probability density function of the random variables X
and Y. [3 marks]
(ii) Marginal density function of X [3 marks]
(iii) Marginal density function of Y [3 marks]
(iv) Conditional density function of X given Y = y. [2 marks]
(v) Conditional density function of Y given X = x. [2 marks]
(vi) Are X and Y independent? [2 marks]
accessedg05 2022-11-28A negative potential energy is possible. Explain
Ramiro Wise 2022-11-28Find the average value fave of the function f on the given interval.
Ada Lloyd 2022-11-27When Sam goes to a restaurant, he always tips the sener $2 plus 10% of the cost of the meal. If Sam's distribution of meal costs has a mean of $9 and a standard deviation of $3, what are the mean and standard deviation of the distribution
Sagar Yadav2022-11-26A customer organization that evaluates new automobiles customarily reports the
number of major defects in each car examined. Let ܺ denote the number of major
defects in a randomly selected car of a certain type. The cdf of ܺ is as follows:
= (ݔ)ܨ
ە
ۖ
ۖ
۔
ۖ
ۖ
ۓ
0 if ݔ > 0
0.06 if 0 ≤ ݔ > 1
0.19 if 1 ≤ ݔ > 2
0.39 if 2 ≤ ݔ > 3
0.67 if 3 ≤ ݔ > 4
0.92 if 4 ≤ ݔ > 5
0.97 if 5 ≤ ݔ > 6
1 if 6 ≤ ݔ
Calculate the following probabilities directly from the cdf.
a. ܲ(ܺ = 2)
b. ܲ(ܺ > 3)
c. ܲ(2 ≤ ܺ ≤ 5)
d. ܲ(2 < ܺ < 5)
A customer organization that evaluates new automobiles customarily reports the
number of major defects in each car examined. Let ܺ denote the number of major
defects in a randomly selected car of a certain type. The cdf of ܺ is as follows:
= (ݔ)ܨ
ە
ۖ
ۖ
۔
ۖ
ۖ
ۓ
0 if ݔ > 0
0.06 if 0 ≤ ݔ > 1
0.19 if 1 ≤ ݔ > 2
0.39 if 2 ≤ ݔ > 3
0.67 if 3 ≤ ݔ > 4
0.92 if 4 ≤ ݔ > 5
0.97 if 5 ≤ ݔ > 6
1 if 6 ≤ ݔ
Calculate the following probabilities directly from the cdf.
a. ܲ(ܺ = 2)
b. ܲ(ܺ > 3)
c. ܲ(2 ≤ ܺ ≤ 5)
d. ܲ(2 < ܺ < 5)
quakbIi 2022-11-26Which of the following quantity remains constant during the uniform circular motion?
