Find the derivative y=\frac{\ln x}{x^6} y=e^{x^5}\ln x Find the indicated derivative of the function. \frac{d^4 y}{dx^4} \text{ of } y=2x^5-3x^2-5x+1
Elleanor Mckenzie
Answered question
2021-05-13
Find the indicated derivative of the function.
Answer & Explanation
pivonie8
Skilled2021-05-14Added 91 answers
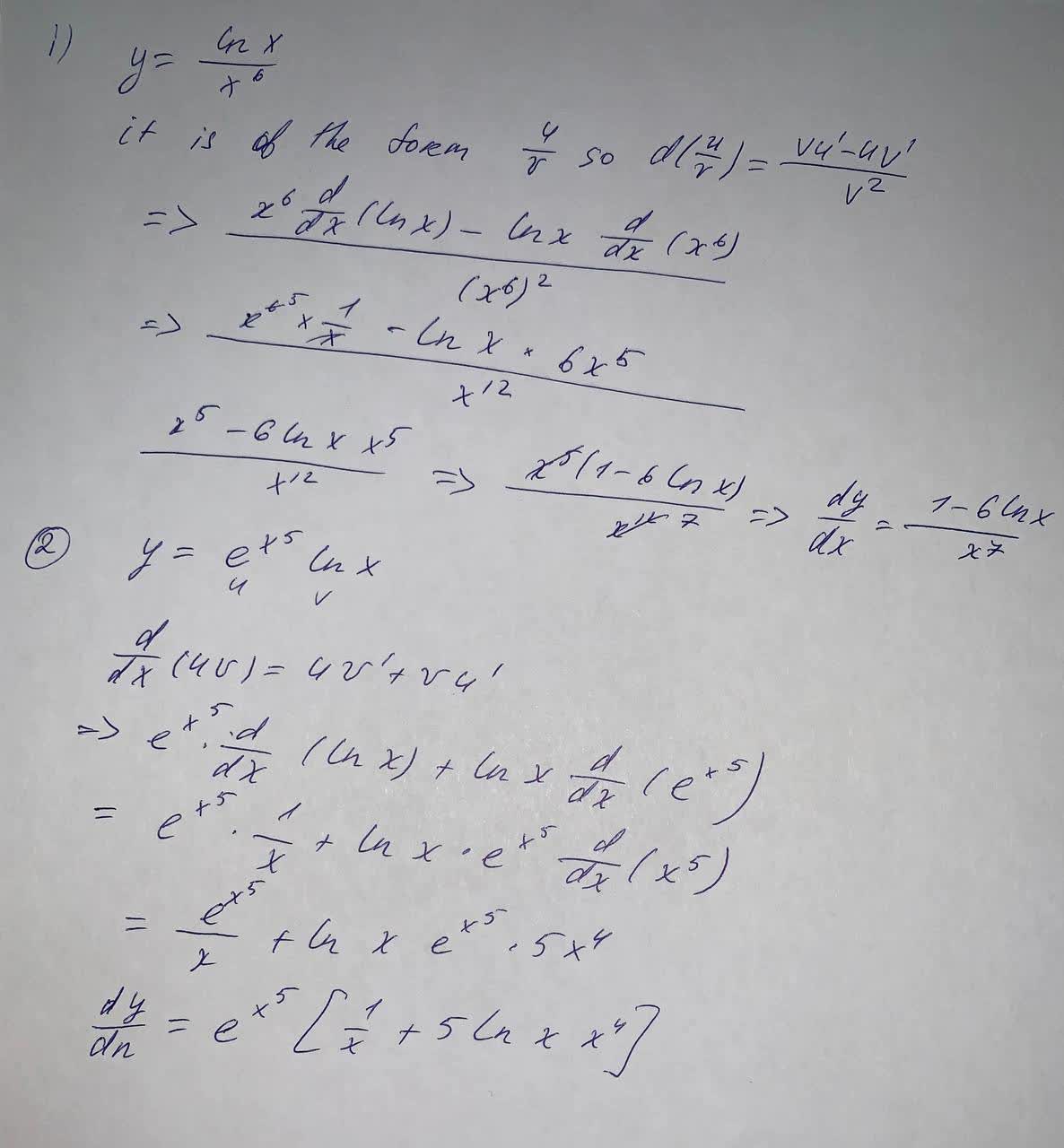

Jeffrey Jordon
Expert2021-09-30Added 2605 answers
1)
it is of the form
2)
Given
Again derivative w.r to x
Again derivative w. r. to x
Again derivative w.r. to x
New Questions in Calculus 1
Find the local maximum and minimum values and saddle points of the function. If you have three-dimensional graphing software, graph the function with a domain and viewpoint that reveal all the important aspects of the function
in derivative?
What is the derivative of ?
What is the limit of as ?
What is the derivative of ?
What is the derivative of ?
How to find as using l'Hospital's Rule?
What is the integral of ?
What is the derivative of ?
What Is the common difference or common ratio of the sequence 2, 5, 8, 11...?
How to find the derivative of ?
How to evaluate the limit as x approaches 0?
How to find derivatives of parametric functions?
What is the antiderivative of ?
How to evaluate: indefinite integral ?