Look at the triangulation of convex polygons again. Prove following statements:Any triangulation of a convex n-polygon subdivides it into n−2 triangles.For example, every triangulation of a convex pentagon has 5−2=3 triangles, and every triangulation of a hexagon has 6−2=4 triangles

Question

Daniella Cochran · Accepted Answer

Step1In the question we have to prove that any triangulation of a convex n-polygon subdivides it into n - 2 triangles.Step2A convex polygon can be defined as a polygon whose interior angles are less than 180°.A triangulation of a convex polygon is given by drawing diagonals between non-adjacent vertices or we cansay corners , also whose diagonal never intersect another diagonal (except at a vertex), until all possible ways of drawing diagonals have been used.For proof of triangulation of a convex n-polygon subdiides it into n- 2 triangles we will sketch polygon of 5and 6 sides and show every possible diagonal.when n=5as we can clearly see there are only 3 triangles possible in a convex pentagon, which proves the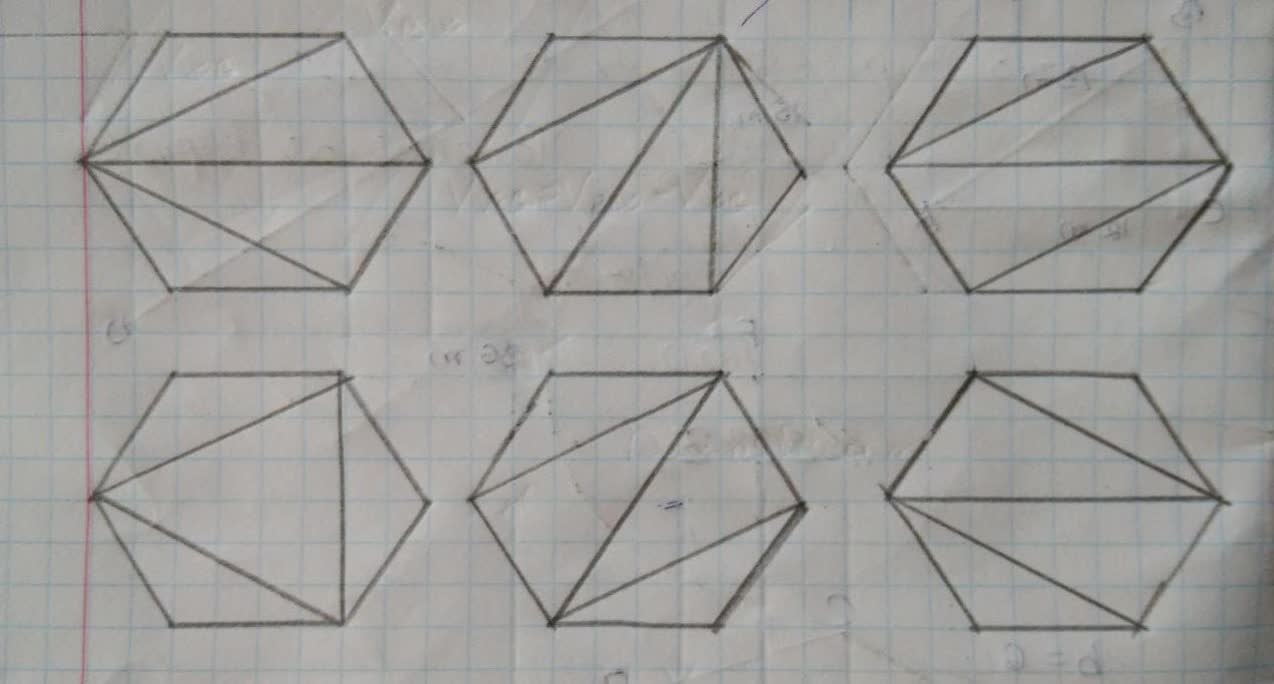statement any triangulation of a convex n-polygon subdivides it into n - 2 triangles.when n=6as we can clearly see there are only 3 triangles possible in a convex hexagon, which proves thestatement any triangulation of a convex n-polygon subdivides it into n - 2 triangles.

Look at the triangulation of convex polygons again. Prove following statements: Any triangulation of a convex n-polygon subdivides it into n - 2 triangles. For example, every triangulation of a convex pentagon has 5 - 2=3 triangles, and every triangulation of a hexagon has 6-2=4 triangles

Open question

Answer & Explanation

New Questions in Trigonometry